Spinodal decomposition of supercritical fluid forms melt network in a silicate-H2O system
Affiliations | Corresponding Author | Cite as | Funding information- Share this article




-
Article views:3,368Cumulative count of HTML views and PDF downloads.
- Download Citation
- Rights & Permissions
top
Abstract
Figures
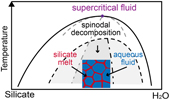
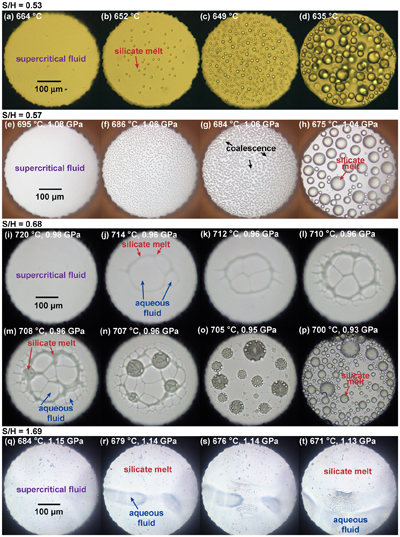 Figure 1 Phenomena observed during cooling and unmixing of supercritical fluid with different silicate to H2O mass ratios (S/H). (a–d) S/H = 0.53, nucleation of silicate melt droplets followed by independent growth. (e–h) S/H = 0.57, spinodal decomposition with homogeneously dispersed silicate melt droplets followed by coalescence. (i–p) S/H = 0.68, spinodal decomposition and development of a silicate melt network, later disrupted into melt droplets. (q–t) S/H = 1.69, spinodal decomposition with large portions of silicate melt and aqueous fluid. | 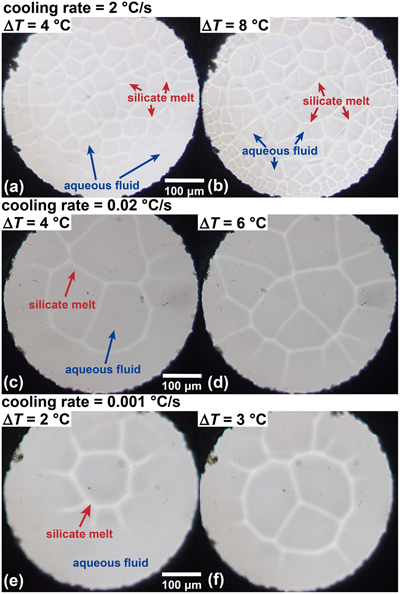 Figure 2 Control of cooling rate on configuration of silicate melt network during spinodal decomposition of supercritical fluid with S/H of 0.92. The ΔT indicates the temperature difference from the point of perceivable unmixing. | 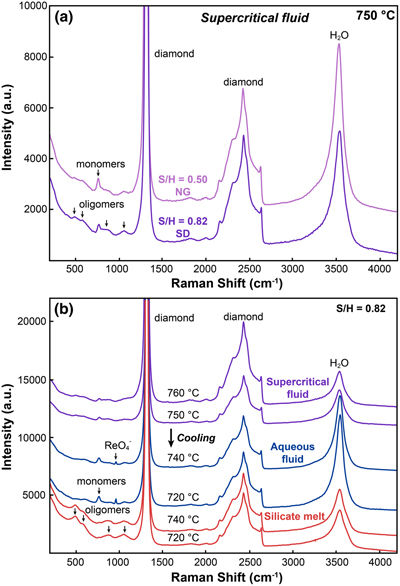 Figure 3 (a) Raman spectra of two supercritical fluids at 750 °C, before unmixing by nucleation-growth (NG with S/H = 0.50, light purple) or by spinodal decomposition (SD with S/H = 0.82, purple), respectively. The S/H = 0.50 fluid contained mainly monomers such as H4SiO4, whereas the S/H = 0.82 fluid contained more oligomers such as H6Si2O7. (b) Raman spectra of the supercritical fluid with S/H = 0.82 and coexisting aqueous fluid and silicate melt formed during cooling. The aqueous fluid contained monomers and ReO4− (formed by oxidation of the Re gasket), whereas the silicate melt contained more oligomers. | 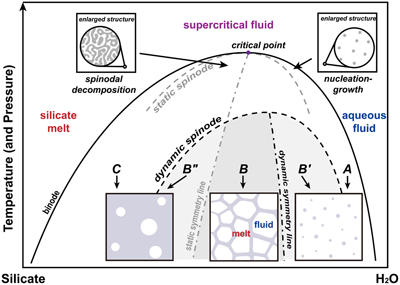 Figure 4 Schematic phase diagram of silicate-H2O systems including dynamic effects. The binode and the static spinode separate the single phase fluid stability field, the metastable field (nucleation-growth field), and the unstable field (spinodal decomposition field), with a static symmetry line marking equal volumes of silicate melt and aqueous fluid. Unmixing starts either by nucleation (composition A and C) or by spinodal decomposition (composition B′, B, and B′′), but is not visible by optical means. Further cooling produces different patterns of melt-fluid distribution controlled by the dynamic spinode and the dynamic symmetry line. For H2O-rich composition B′, dispersed melt droplets (Fig. 1e–h) are formed, optically similar to composition A (Fig. 1a–d). For silicate-rich composition B′′, aqueous fluid droplets (Fig. 1q–t) appear, optically similar to composition C. For composition B between the two symmetry lines, a network of silicate melt can be stabilised by elastic stress, before being disintegrated into dispersed melt droplets by interfacial tension (Fig. 1i–p). Explanations are partly based on the theory of viscoelastic phase separation (Tanaka, 1994). |
| Figure 1 | Figure 2 | Figure 3 | Figure 4 |
top
Introduction
With increasing pressure and temperature in Earth’s interior, the miscibility gap between silicate melt and aqueous fluid diminishes, and single phase supercritical fluid with an intermediate composition (both silicate solute and H2O are in the range of 30–70 wt. %) can form, most likely in deep subduction zones (Manning, 2004
Manning, C.E. (2004) The chemistry of subduction zone fluids. Earth and Planetary Science Letters 233, 1–16.
; Frezzotti and Ferrando, 2015Frezzotti, M.L., Ferrando, S. (2015) The chemical behaviour of fluids released during deep subduction based on fluid inclusions. American Mineralogist 100, 352–377.
; Ni et al., 2017Ni, H., Zhang, L., Xiong, X., Mao, Z., Wang, J. (2017) Supercritical fluids at subduction zones: Evidence, formation condition, and physicochemical properties. Earth-Science Reviews 167, 62–71.
) and in pegmatite systems (Sowerby and Keppler, 2002Sowerby, J.R., Keppler, H. (2002) The effect of fluorine, boron and excess sodium on the critical curve in the albite–H2O system. Contributions to Mineralogy and Petrology 143, 32–37.
). Supercritical fluid can be an important agent of mass transfer because it can carry large amounts of elements (Kessel et al., 2005Kessel, R., Schmidt, M.W., Ulmer, P., Pettke, T. (2005) Trace element signature of subduction-zone fluids, melts and supercritical liquids at 120–180 km depth. Nature 437, 724–727.
; Schmidt and Poli, 2014Schmidt, M.W., Poli, S. (2014) 4.19 - Devolatilisation during subduction. In: Holland, H.D., Turekian, K.K. (Eds.) Treatise on Geochemistry. Second Edition, Elsevier, Amsterdam, 669–701.
) while keeping low viscosity (Audétat and Keppler, 2004Audétat, A., Keppler, H. (2004) Viscosity of fluids in subduction zones. Science 303, 513–516.
). The stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997Shen, A.H., Keppler, H. (1997) Direct observation of complete miscibility in the albite–H2O system. Nature 385, 68–77.
; Bureau and Keppler, 1999Bureau, H., Keppler, H. (1999) Complete miscibility between silicate melts and hydrous fluids in the upper mantle: experimental evidence and geochemical implications. Earth and Planetary Science Letters 165, 187–196.
; Sowerby and Keppler, 2002Sowerby, J.R., Keppler, H. (2002) The effect of fluorine, boron and excess sodium on the critical curve in the albite–H2O system. Contributions to Mineralogy and Petrology 143, 32–37.
; Mibe et al., 2007Mibe, K., Kanzaki, M., Kawamoto, T., Matsukage, K.N., Fei, Y., Ono, S. (2007) Second critical endpoint in the peridotite-H2O system. Journal of Geophysical Research 112, B03201.
, 2008Mibe, K., Chou, I.M., Bassett, W.A. (2008) In situ Raman spectroscopic investigation of the structure of subduction-zone fluids. Journal of Geophysical Research 113, B04208.
, 2011Mibe, K., Kawamoto, T., Matsukage, K.N., Fei, Y., Ono, S. (2011) Slab melting versus slab dehydration in subduction-zone magmatism. Proceedings of the National Academy of Sciences 108, 8177–8182.
). Coexisting melt and fluid inclusions with a wide range of silicate to H2O ratios in pegmatites (Zajacz et al., 2008Zajacz, Z., Halter, W., Pettke, T., Guillong, M. (2008) Determination of fluid/melt partition coefficients by LA-ICPMS analysis of co-existing fluid and silicate melt inclusions: Controls on element partitioning. Geochimica et Cosmochimica Acta 72, 2169–2197.
) imply that decreasing temperature and pressure (to <700 °C and <1 GPa) can drive supercritical fluid to a miscibility gap, and cause its unmixing into coexisting melt and fluid. Unmixing of supercritical fluid is important for unravelling fluid differentiation and melt/fluid inclusion records in both subduction zone and pegmatite settings, but this unmixing process has not been well documented and understood (all previous experiments focused on mixing of silicate and H2O and the locus of critical curves). Here, we report some novel results from direct observation of unmixing of supercritical fluid in a silicate-H2O system at conditions pertinent to peralkaline aluminosilicate systems.top
Methods
Experiments (see Supplementary Information for details) were carried out in a Bassett-type hydrothermal diamond anvil cell (HDAC) (Bassett et al., 1993
Bassett, W.A., Shen, A.H., Bucknum, M. (1993) A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from −190 to 1200 °C. Review of Scientific Instruments 64, 2340.
). Sample chamber was initially filled with a piece of sodium aluminosilicate glass (Na3AlSi5O13 with 1.5 wt. % H2O), distilled water, and an air bubble, with the mass ratio of silicate to H2O (S/H) ranging from 0.47–1.69 (32 to 63 wt. % silicate; Table S-1). This peralkaline silicate composition was chosen because its complete miscibility does not require extremely high pressure and temperature that would pose serious challenges to HDAC. This heterogeneous solid-liquid-gas system was homogenised into a supercritical fluid upon heating to ∼720 °C and concomitant pressurisation to ∼1 GPa. During cooling to ∼650 °C with cooling rates of 0.001–2 °C/s, unmixing of supercritical fluid was observed by an Olympus BX53 optical microscope with 20× magnification objective (numerical aperture = 0.25) equipped with a camera recorder. Raman spectra of supercritical fluids, silicate melts and aqueous fluids were collected in situ with a Horiba Jobin-Yvon LabRam HR Evolution spectrometer.top
Results
A summary of experimental conditions and results is presented in Table S-1, and the videos of selected experiments are available in Supplementary Information. When a supercritical fluid was subjected to cooling, a variety of phenomena were observed depending on the S/H ratio of the Na3AlSi5O13-H2O system. For S/H less than 0.54, silicate melt droplets were nucleated at discrete sites (Fig. 1a,b), followed by droplet growth and coalescence (Fig. 1c,d). When S/H increased to ∼0.57, silicate melt droplets formed homogeneously with a high number density (Fig. 1e–h), and the coalescence of droplets became more prominent. For greater S/H such as 0.68, a spectacular network of silicate melt, with multiple threads and nodes, developed during cooling (Fig. 1i–l). Increasing image contrast between silicate melt network and aqueous fluid (both phases are continuous) reflected increasing difference in their composition. Melt subsequently retreated toward a number of nodes (Fig. 1m,n), leading to disruption to the network (Fig. 1o). The bicontinuous texture was converted to melt droplets dispersed in the fluid (Fig. 1p). With an increase of S/H from 0.58 to 1.05 (37 to 51 wt. % silicate), melt threads became stronger, alongside less congregation of melt at the nodes (Fig. S-2). At the highest S/H of 1.69 being investigated, instead of a melt network we observed large pockets of aqueous fluid (Fig. 1q–t).

Figure 1 Phenomena observed during cooling and unmixing of supercritical fluid with different silicate to H2O mass ratios (S/H). (a–d) S/H = 0.53, nucleation of silicate melt droplets followed by independent growth. (e–h) S/H = 0.57, spinodal decomposition with homogeneously dispersed silicate melt droplets followed by coalescence. (i–p) S/H = 0.68, spinodal decomposition and development of a silicate melt network, later disrupted into melt droplets. (q–t) S/H = 1.69, spinodal decomposition with large portions of silicate melt and aqueous fluid.
For experimentally practical cooling rates, a silicate melt network always formed as long as the S/H was in the intermediate range of 0.58 to 1.05. When the cooling rate decreased from 2 °C/s to 0.02 °C/s, melt network became sparse, and the size of domains (mainly as pentagonal and hexagonal sections in 2D) increased correspondingly (Fig. 2a–d). Melt network was fairly stable – when cooling rate decreased to 0.001 °C/s, melt network could persist for more than 60 min (Fig. 2e,f). The reversibility of texture evolution is also worth noting: upon heating, dispersed melt droplets reconnected into a network before homogenisation into a supercritical fluid occurred.

Figure 2 Control of cooling rate on configuration of silicate melt network during spinodal decomposition of supercritical fluid with S/H of 0.92. The ΔT indicates the temperature difference from the point of perceivable unmixing.
Raman spectra of supercritical fluids, silicate melts, and aqueous fluids are presented in Figure 3. For supercritical fluid (Fig. 3a), the band at ∼770 cm−1, assigned to the vibration of silica monomers (Zotov and Keppler, 2000
Zotov, N., Keppler, H. (2000) In-situ Raman spectra of dissolved silica species in aqueous fluids to 900 °C and 14 kbar. American Mineralogist 85, 600–604.
), weakened with an increase of S/H from 0.5 to 0.82. Concomitantly, the intensities of the broad bands at 400–700 cm−1, 790–960 cm−1 and ∼1050 cm−1 (Fig. 3a), related to polymerised aluminosilicate species (i.e. dimers and trimers) (Mibe et al., 2008Mibe, K., Chou, I.M., Bassett, W.A. (2008) In situ Raman spectroscopic investigation of the structure of subduction-zone fluids. Journal of Geophysical Research 113, B04208.
; Dalou et al., 2014Dalou, C., Mysen, B.O., Foustoukos, D. (2014) In-situ measurements of fluorine and chlorine speciation and partitioning between melts and aqueous fluids in the Na2O-Al2O3-SiO2-H2O system. American Mineralogist 100, 47–58.
), appeared to increase. After unmixing of supercritical fluid with S/H = 0.82, the 770 cm−1 band in the spectra of aqueous fluids indicated significant presence of monomers (Fig. 3b). By contrast, the spectra of silicate melts suggested the dominance of polymerised aluminosilicate species.
Figure 3 (a) Raman spectra of two supercritical fluids at 750 °C, before unmixing by nucleation-growth (NG with S/H = 0.50, light purple) or by spinodal decomposition (SD with S/H = 0.82, purple), respectively. The S/H = 0.50 fluid contained mainly monomers such as H4SiO4, whereas the S/H = 0.82 fluid contained more oligomers such as H6Si2O7. (b) Raman spectra of the supercritical fluid with S/H = 0.82 and coexisting aqueous fluid and silicate melt formed during cooling. The aqueous fluid contained monomers and ReO4− (formed by oxidation of the Re gasket), whereas the silicate melt contained more oligomers.
top
Discussion
Experimental results demonstrate the primary control of bulk composition, as indexed by the S/H ratio, over the mechanism and process of unmixing of supercritical fluid in silicate-H2O systems (Fig. 4). A H2O-rich supercritical fluid with S/H = ∼0.5 (composition A in Fig. 4) contains predominantly Si and Al monomers (Fig. 3a). Assembling these monomers to clusters would exhaust silicate solute in the local environment, leading to nucleation of silicate melt droplets at discrete sites (Fig. 1a–d). This nucleation-growth mechanism needs to overcome interfacial energy, and is essentially similar to the exsolution of fluid bubbles from a hydrous magma (composition C in Fig. 4), except that the roles of silicate and H2O are exchanged.

Figure 4 Schematic phase diagram of silicate-H2O systems including dynamic effects. The binode and the static spinode separate the single phase fluid stability field, the metastable field (nucleation-growth field), and the unstable field (spinodal decomposition field), with a static symmetry line marking equal volumes of silicate melt and aqueous fluid. Unmixing starts either by nucleation (composition A and C) or by spinodal decomposition (composition B′, B, and B′′), but is not visible by optical means. Further cooling produces different patterns of melt-fluid distribution controlled by the dynamic spinode and the dynamic symmetry line. For H2O-rich composition B′, dispersed melt droplets (Fig. 1e–h) are formed, optically similar to composition A (Fig. 1a–d). For silicate-rich composition B′′, aqueous fluid droplets (Fig. 1q–t) appear, optically similar to composition C. For composition B between the two symmetry lines, a network of silicate melt can be stabilised by elastic stress, before being disintegrated into dispersed melt droplets by interfacial tension (Fig. 1i–p). Explanations are partly based on the theory of viscoelastic phase separation (Tanaka, 1994
Tanaka, H. (1994) Critical dynamics and phase-separation kinetics in dynamically asymmetric binary fluids: New dynamic universality class for polymer mixtures or dynamic crossover? The Journal of Chemical Physics 100, 5323.
).By contrast, the observed melt network for S/H in the range of 0.58–1.05, was unlikely to be formed by nucleation-growth, but probably derived from an early stage of spinodal decomposition throughout the supercritical fluid. According to theories of thermodynamics and phase separation (Cahn, 1965
Cahn, J.W. (1965) Phase separation by spinodal decomposition in isotropic system. The Journal of Chemical Physics 42, 93–99.
; Debenedetti, 2000Debenedetti, P.G. (2000) Phase separation by nucleation and by spinodal decomposition: fundamentals. In: Kiran, E., Debenedetti, P.G., Peters, C.J. (Eds.) Supercritical Fluids. Nato Science Series E, Volume 366. Springer, Dordrecht, 123–166.
), when a homogeneous solution falls between the binode (solvus) and the spinode of a miscibility gap, it would either stay metastable or unmix by nucleating a daughter phase with a different composition. But when the solution falls inside the spinode, concentration fluctuation would lead to spontaneous and uniform unmixing and form a bicontinuous structure with high phase interconnectivity, referred to as spinodal decomposition. The presence of polymerised Si-Al-Na species (Fig. 3a) could facilitate spinodal decomposition of supercritical fluid.The initial stage of spinodal decomposition was difficult to capture by optical microscope due to the limit on spatial resolution (no better than 2 μm). For S/H = 0.54–0.57 (composition B´ in Fig. 4), the observation of uniformly distributed melt droplets implies inheritance from spinodal decomposition. Stronger evidence of spinodal decomposition, however, still came from the observation of melt network (composition B in Fig. 4), as explained by the theory of viscoelastic phase separation (VPS) for polymer solutions (Tanaka, 1994
Tanaka, H. (1994) Critical dynamics and phase-separation kinetics in dynamically asymmetric binary fluids: New dynamic universality class for polymer mixtures or dynamic crossover? The Journal of Chemical Physics 100, 5323.
; Iwashita and Tanaka, 2006Iwashita, Y., Tanaka, H. (2006) Self-organisation in phase separation of a lyotropic liquid crystal into cellular, network and droplet morphologies. Nature Materials 5, 147–152.
).According to the VPS theory, contrasting dynamics between two components of a binary solution is key to the formation of a network pattern. In a supercritical fluid, the silicate component is polymerised and involves a long relaxation time, whereas H2O is a small molecule and dynamically fast. This dynamic asymmetry led to a large difference in viscoelastic properties between coexisting silicate melt and aqueous fluid, and a melt network was stabilised by elastic stress induced by the slower silicate component. The approximately 120° angle between silicate melt threads (Fig. 1j–n) supports the existence of stress. For geologically relevant cooling rates of magmatic-hydrothermal systems (of the order of °C/kyr to °C/Myr; Lake, 2013
Lake, E.T. (2013) Crystallisation and saturation front propagation in silicic magma chambers. Earth and Planetary Science Letters 383, 182–193.
), which are much lower than our experimental values (0.001–2 °C/s), a silicate melt network is expected to be highly stable.Further cooling enlarged the compositional difference between silicate melt and aqueous fluid and hence interfacial tension. After outweighing the elastic stress, interfacial tension eventually caused disintegration of the melt network, and the structure was relaxed into dispersed melt droplets. In principle, the relaxation rate should be controlled by interfacial tension and melt viscosity (Tanaka and Araki, 2006
Tanaka, H., Araki, T. (2006) Viscoelastic phase separation in soft matter: Numerical-simulation study on its physical mechanism. Chemical Engineering Science 61, 2108–2141.
), although we still lack the essential parameters to verify this relationship quantitatively.Tanaka (1994)
Tanaka, H. (1994) Critical dynamics and phase-separation kinetics in dynamically asymmetric binary fluids: New dynamic universality class for polymer mixtures or dynamic crossover? The Journal of Chemical Physics 100, 5323.
suggested the presence of a dynamic spinode inside the thermodynamic (static) spinode (Fig. 4). A network pattern appears in the region between the static symmetry line (corresponding to equal volumes of silicate melt and aqueous fluid) and the dynamic symmetry line. Both unmixing mechanisms, spinodal decomposition and nucleation-growth, can produce droplets structure, but a melt network is unique to viscoelastic spinodal decomposition involving dynamic asymmetry.The development of a stable silicate melt network during unmixing of supercritical fluid can have important implications for magmatic-hydrothermal systems. Some granitic pegmatites contain fluid/melt inclusions with a spectrum of silicate to H2O ratios (Zajacz et al., 2008
Zajacz, Z., Halter, W., Pettke, T., Guillong, M. (2008) Determination of fluid/melt partition coefficients by LA-ICPMS analysis of co-existing fluid and silicate melt inclusions: Controls on element partitioning. Geochimica et Cosmochimica Acta 72, 2169–2197.
; Thomas et al., 2012Thomas, R., Davidson, P., Beurlen, H. (2012) The competing models for the origin and internal evolution of granitic pegmatites in the light of melt and fluid inclusion research. Mineralogy and Petrology 106, 55–73.
), which could be attributed to heterogeneous entrapment of coexisting silicate melt and aqueous fluid. Compared to a droplets structure with smooth spherical fluid-melt phase boundaries, a silicate melt network with uneven phase boundaries would increase the likelihood of trapping melt and fluid together in different proportions. Furthermore, unmixing by spinodal decomposition and formation of an extensive melt network, in contrast to nucleation of fluid/melt droplets at discrete sites, can promote the efficiency of separating aqueous (hydrothermal) fluid from silicate melt. This, in turn, can contribute to the extraction of metals by hydrothermal fluid, and hence to mineralisation.top
Conclusions
Our experimental observations demonstrate that the mechanism and process of unmixing of supercritical fluid in silicate-H2O systems are primarily controlled by its composition. Spinodal decomposition of supercritical fluid with 37 to 51 wt. % silicate developed into a stable silicate melt network due to elastic stress supported by the polymerised silicate component. The occurrence of silicate melt network could facilitate heterogeneous entrapment of coexisting silicate melt and aqueous fluid, and promote the efficiency of melt-fluid separation and mineralisation in magmatic-hydrothermal systems.
top
Acknowledgements
Instructive comments from Alexander P. Gysi and an anonymous reviewer significantly improved the manuscript. Discussion with Hans Keppler and Youxue Zhang was beneficial. This work was supported by the National Key R&D Programme of China (2018YFA0702700), the National Natural Science Foundation of China (41825004, 41721002), and the Fundamental Research Funds for the Central Universities of China (WK3410000013).
Editor: Horst R. Marschall
top
References
Audétat, A., Keppler, H. (2004) Viscosity of fluids in subduction zones. Science 303, 513–516.
 Show in context
Show in contextSupercritical fluid can be an important agent of mass transfer because it can carry large amounts of elements (Kessel et al., 2005; Schmidt and Poli, 2014) while keeping low viscosity (Audétat and Keppler, 2004).
View in article
Bassett, W.A., Shen, A.H., Bucknum, M. (1993) A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from −190 to 1200 °C. Review of Scientific Instruments 64, 2340.
 Show in context
Show in contextExperiments (see Supplementary Information for details) were carried out in a Bassett-type hydrothermal diamond anvil cell (HDAC) (Bassett et al., 1993).
View in article
Bureau, H., Keppler, H. (1999) Complete miscibility between silicate melts and hydrous fluids in the upper mantle: experimental evidence and geochemical implications. Earth and Planetary Science Letters 165, 187–196.
 Show in context
Show in contextThe stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
Cahn, J.W. (1965) Phase separation by spinodal decomposition in isotropic system. The Journal of Chemical Physics 42, 93–99.
 Show in context
Show in contextAccording to theories of thermodynamics and phase separation (Cahn, 1965; Debenedetti, 2000), when a homogeneous solution falls between the binode (solvus) and the spinode of a miscibility gap, it would either stay metastable or unmix by nucleating a daughter phase with a different composition.
View in article
Dalou, C., Mysen, B.O., Foustoukos, D. (2014) In-situ measurements of fluorine and chlorine speciation and partitioning between melts and aqueous fluids in the Na2O-Al2O3-SiO2-H2O system. American Mineralogist 100, 47–58.
 Show in context
Show in contextConcomitantly, the intensities of the broad bands at 400–700 cm−1, 790–960 cm−1 and ∼1050 cm−1 (Fig. 3a), related to polymerised aluminosilicate species (i.e. dimers and trimers) (Mibe et al., 2008; Dalou et al., 2014), appeared to increase.
View in article
Debenedetti, P.G. (2000) Phase separation by nucleation and by spinodal decomposition: fundamentals. In: Kiran, E., Debenedetti, P.G., Peters, C.J. (Eds.) Supercritical Fluids. Nato Science Series E, Volume 366. Springer, Dordrecht, 123–166.
 Show in context
Show in contextAccording to theories of thermodynamics and phase separation (Cahn, 1965; Debenedetti, 2000), when a homogeneous solution falls between the binode (solvus) and the spinode of a miscibility gap, it would either stay metastable or unmix by nucleating a daughter phase with a different composition.
View in article
Frezzotti, M.L., Ferrando, S. (2015) The chemical behaviour of fluids released during deep subduction based on fluid inclusions. American Mineralogist 100, 352–377.
 Show in context
Show in contextWith increasing pressure and temperature in Earth’s interior, the miscibility gap between silicate melt and aqueous fluid diminishes, and single phase supercritical fluid with an intermediate composition (both silicate solute and H2O are in the range of 30–70 wt. %) can form, most likely in deep subduction zones (Manning, 2004; Frezzotti and Ferrando, 2015; Ni et al., 2017) and in pegmatite systems (Sowerby and Keppler, 2002).
View in article
Iwashita, Y., Tanaka, H. (2006) Self-organisation in phase separation of a lyotropic liquid crystal into cellular, network and droplet morphologies. Nature Materials 5, 147–152.
 Show in context
Show in contextStronger evidence of spinodal decomposition, however, still came from the observation of melt network (composition B in Fig. 4), as explained by the theory of viscoelastic phase separation (VPS) for polymer solutions (Tanaka, 1994; Iwashita and Tanaka, 2006).
View in article
Kessel, R., Schmidt, M.W., Ulmer, P., Pettke, T. (2005) Trace element signature of subduction-zone fluids, melts and supercritical liquids at 120–180 km depth. Nature 437, 724–727.
 Show in context
Show in contextSupercritical fluid can be an important agent of mass transfer because it can carry large amounts of elements (Kessel et al., 2005; Schmidt and Poli, 2014) while keeping low viscosity (Audétat and Keppler, 2004).
View in article
Lake, E.T. (2013) Crystallisation and saturation front propagation in silicic magma chambers. Earth and Planetary Science Letters 383, 182–193.
 Show in context
Show in contextFor geologically relevant cooling rates of magmatic-hydrothermal systems (of the order of °C/kyr to °C/Myr; Lake, 2013), which are much lower than our experimental values (0.001–2 °C/s), a silicate melt network is expected to be highly stable.
View in article
Manning, C.E. (2004) The chemistry of subduction zone fluids. Earth and Planetary Science Letters 233, 1–16.
 Show in context
Show in contextWith increasing pressure and temperature in Earth’s interior, the miscibility gap between silicate melt and aqueous fluid diminishes, and single phase supercritical fluid with an intermediate composition (both silicate solute and H2O are in the range of 30–70 wt. %) can form, most likely in deep subduction zones (Manning, 2004; Frezzotti and Ferrando, 2015; Ni et al., 2017) and in pegmatite systems (Sowerby and Keppler, 2002).
View in article
Mibe, K., Kanzaki, M., Kawamoto, T., Matsukage, K.N., Fei, Y., Ono, S. (2007) Second critical endpoint in the peridotite-H2O system. Journal of Geophysical Research 112, B03201.
 Show in context
Show in contextThe stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
Mibe, K., Chou, I.M., Bassett, W.A. (2008) In situ Raman spectroscopic investigation of the structure of subduction-zone fluids. Journal of Geophysical Research 113, B04208.
 Show in context
Show in contextConcomitantly, the intensities of the broad bands at 400–700 cm−1, 790–960 cm−1 and ∼1050 cm−1 (Fig. 3a), related to polymerised aluminosilicate species (i.e. dimers and trimers) (Mibe et al., 2008; Dalou et al., 2014), appeared to increase.
View in article
The stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
Mibe, K., Kawamoto, T., Matsukage, K.N., Fei, Y., Ono, S. (2011) Slab melting versus slab dehydration in subduction-zone magmatism. Proceedings of the National Academy of Sciences 108, 8177–8182.
 Show in context
Show in context
The stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
Ni, H., Zhang, L., Xiong, X., Mao, Z., Wang, J. (2017) Supercritical fluids at subduction zones: Evidence, formation condition, and physicochemical properties. Earth-Science Reviews 167, 62–71.
 Show in context
Show in contextWith increasing pressure and temperature in Earth’s interior, the miscibility gap between silicate melt and aqueous fluid diminishes, and single phase supercritical fluid with an intermediate composition (both silicate solute and H2O are in the range of 30–70 wt. %) can form, most likely in deep subduction zones (Manning, 2004; Frezzotti and Ferrando, 2015; Ni et al., 2017) and in pegmatite systems (Sowerby and Keppler, 2002).
View in article
Schmidt, M.W., Poli, S. (2014) 4.19 - Devolatilisation during subduction. In: Holland, H.D., Turekian, K.K. (Eds.) Treatise on Geochemistry. Second Edition, Elsevier, Amsterdam, 669–701.
 Show in context
Show in contextSupercritical fluid can be an important agent of mass transfer because it can carry large amounts of elements (Kessel et al., 2005; Schmidt and Poli, 2014) while keeping low viscosity (Audétat and Keppler, 2004).
View in article
Shen, A.H., Keppler, H. (1997) Direct observation of complete miscibility in the albite–H2O system. Nature 385, 68–77.
 Show in context
Show in contextThe stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
Sowerby, J.R., Keppler, H. (2002) The effect of fluorine, boron and excess sodium on the critical curve in the albite–H2O system. Contributions to Mineralogy and Petrology 143, 32–37.
 Show in context
Show in contextThe stability field of supercritical fluids is largely determined by the critical curves of silicate-H2O systems, which were found by experiments to be generally above 1 GPa with negative P-T slopes (Shen and Keppler, 1997; Bureau and Keppler, 1999; Sowerby and Keppler, 2002; Mibe et al., 2007, 2008, 2011).
View in article
With increasing pressure and temperature in Earth’s interior, the miscibility gap between silicate melt and aqueous fluid diminishes, and single phase supercritical fluid with an intermediate composition (both silicate solute and H2O are in the range of 30–70 wt. %) can form, most likely in deep subduction zones (Manning, 2004; Frezzotti and Ferrando, 2015; Ni et al., 2017) and in pegmatite systems (Sowerby and Keppler, 2002).
View in article
Tanaka, H. (1994) Critical dynamics and phase-separation kinetics in dynamically asymmetric binary fluids: New dynamic universality class for polymer mixtures or dynamic crossover? The Journal of Chemical Physics 100, 5323.
 Show in context
Show in contextExplanations are partly based on the theory of viscoelastic phase separation (Tanaka, 1994).
View in article
Tanaka (1994) suggested the presence of a dynamic spinode inside the thermodynamic (static) spinode (Fig. 4).
View in article
Stronger evidence of spinodal decomposition, however, still came from the observation of melt network (composition B in Fig. 4), as explained by the theory of viscoelastic phase separation (VPS) for polymer solutions (Tanaka, 1994; Iwashita and Tanaka, 2006).
View in article
Tanaka, H., Araki, T. (2006) Viscoelastic phase separation in soft matter: Numerical-simulation study on its physical mechanism. Chemical Engineering Science 61, 2108–2141.
 Show in context
Show in contextIn principle, the relaxation rate should be controlled by interfacial tension and melt viscosity (Tanaka and Araki, 2006), although we still lack the essential parameters to verify this relationship quantitatively.
View in article
Thomas, R., Davidson, P., Beurlen, H. (2012) The competing models for the origin and internal evolution of granitic pegmatites in the light of melt and fluid inclusion research. Mineralogy and Petrology 106, 55–73.
 Show in context
Show in contextSome granitic pegmatites contain fluid/melt inclusions with a spectrum of silicate to H2O ratios (Zajacz et al., 2008; Thomas et al., 2012), which could be attributed to heterogeneous entrapment of coexisting silicate melt and aqueous fluid.
View in article
Zajacz, Z., Halter, W., Pettke, T., Guillong, M. (2008) Determination of fluid/melt partition coefficients by LA-ICPMS analysis of co-existing fluid and silicate melt inclusions: Controls on element partitioning. Geochimica et Cosmochimica Acta 72, 2169–2197.
 Show in context
Show in contextCoexisting melt and fluid inclusions with a wide range of silicate to H2O ratios in pegmatites (Zajacz et al., 2008) imply that decreasing temperature and pressure (to <700 °C and <1 GPa) can drive supercritical fluid to a miscibility gap, and cause its unmixing into coexisting melt and fluid.
View in article
Some granitic pegmatites contain fluid/melt inclusions with a spectrum of silicate to H2O ratios (Zajacz et al., 2008; Thomas et al., 2012), which could be attributed to heterogeneous entrapment of coexisting silicate melt and aqueous fluid.
View in article
Zotov, N., Keppler, H. (2000) In-situ Raman spectra of dissolved silica species in aqueous fluids to 900 °C and 14 kbar. American Mineralogist 85, 600–604.
 Show in context
Show in contextFor supercritical fluid (Fig. 3a), the band at ∼770 cm−1, assigned to the vibration of silica monomers (Zotov and Keppler, 2000), weakened with an increase of S/H from 0.5 to 0.82.
View in article
top
Supplementary Information
The Supplementary Information includes:
- Materials and Methods
- Table S-1
- Figures S-1 and S-2
- Videos S-1 to S-8
- Supplementary Information References
Download Video S-1 (GIF).
Download Video S-2 (GIF).
Download Video S-3 (GIF).
Download Video S-4 (GIF).
Download Video S-5 (GIF).
Download Video S-6 (GIF).
Download Video S-7 (GIF).
Download Video S-8 (GIF).
Download the Supplementary Information (PDF).
Figures
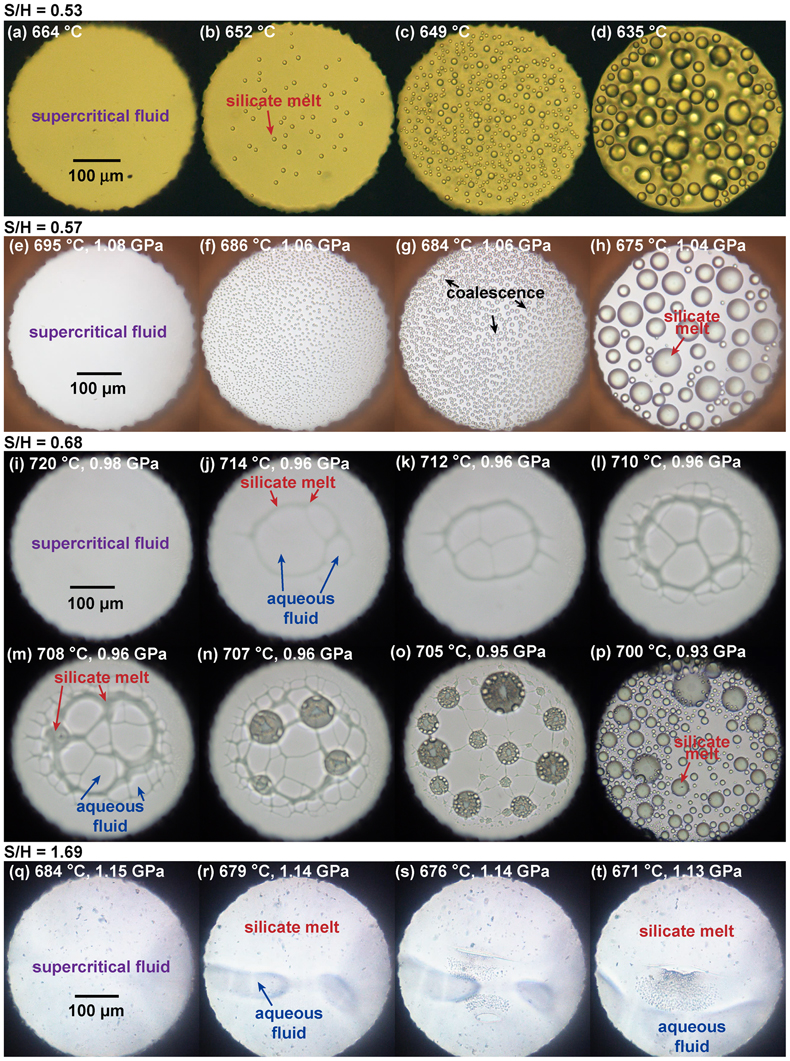
Figure 1 Phenomena observed during cooling and unmixing of supercritical fluid with different silicate to H2O mass ratios (S/H). (a–d) S/H = 0.53, nucleation of silicate melt droplets followed by independent growth. (e–h) S/H = 0.57, spinodal decomposition with homogeneously dispersed silicate melt droplets followed by coalescence. (i–p) S/H = 0.68, spinodal decomposition and development of a silicate melt network, later disrupted into melt droplets. (q–t) S/H = 1.69, spinodal decomposition with large portions of silicate melt and aqueous fluid.
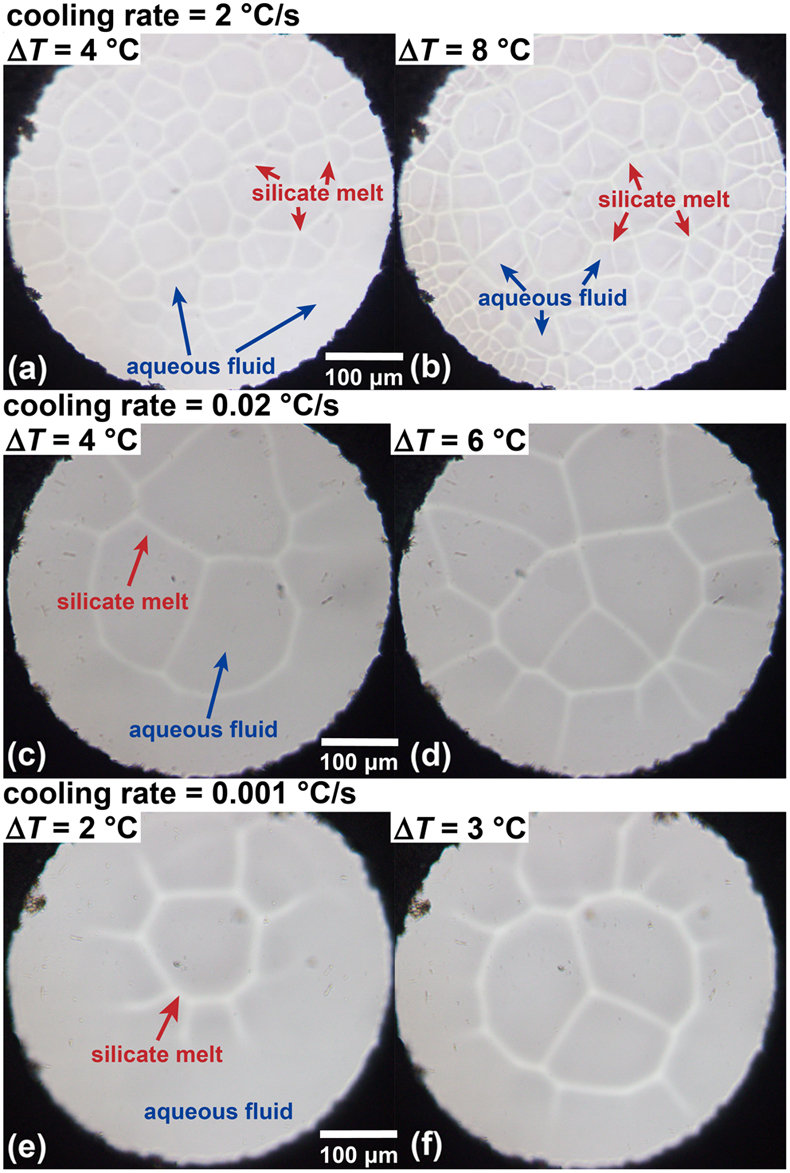
Figure 2 Control of cooling rate on configuration of silicate melt network during spinodal decomposition of supercritical fluid with S/H of 0.92. The ΔT indicates the temperature difference from the point of perceivable unmixing.

Figure 3 (a) Raman spectra of two supercritical fluids at 750 °C, before unmixing by nucleation-growth (NG with S/H = 0.50, light purple) or by spinodal decomposition (SD with S/H = 0.82, purple), respectively. The S/H = 0.50 fluid contained mainly monomers such as H4SiO4, whereas the S/H = 0.82 fluid contained more oligomers such as H6Si2O7. (b) Raman spectra of the supercritical fluid with S/H = 0.82 and coexisting aqueous fluid and silicate melt formed during cooling. The aqueous fluid contained monomers and ReO4− (formed by oxidation of the Re gasket), whereas the silicate melt contained more oligomers.
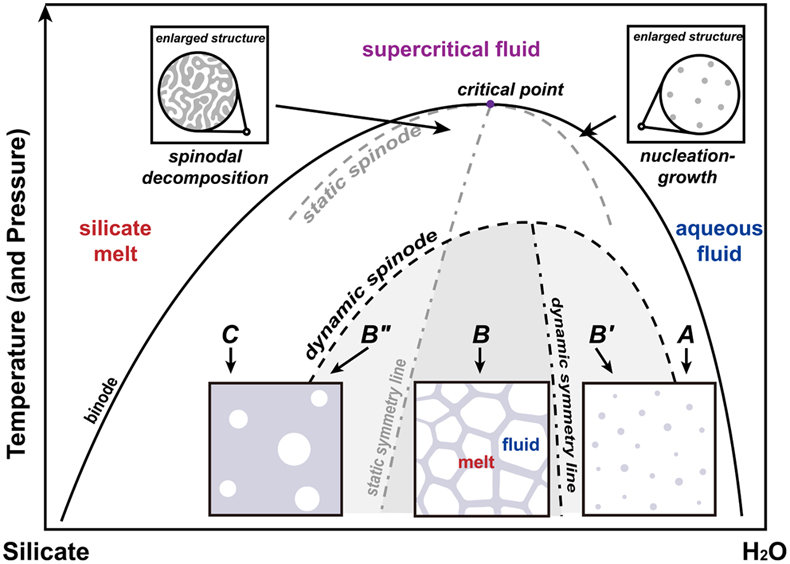
Figure 4 Schematic phase diagram of silicate-H2O systems including dynamic effects. The binode and the static spinode separate the single phase fluid stability field, the metastable field (nucleation-growth field), and the unstable field (spinodal decomposition field), with a static symmetry line marking equal volumes of silicate melt and aqueous fluid. Unmixing starts either by nucleation (composition A and C) or by spinodal decomposition (composition B′, B, and B′′), but is not visible by optical means. Further cooling produces different patterns of melt-fluid distribution controlled by the dynamic spinode and the dynamic symmetry line. For H2O-rich composition B′, dispersed melt droplets (Fig. 1e–h) are formed, optically similar to composition A (Fig. 1a–d). For silicate-rich composition B′′, aqueous fluid droplets (Fig. 1q–t) appear, optically similar to composition C. For composition B between the two symmetry lines, a network of silicate melt can be stabilised by elastic stress, before being disintegrated into dispersed melt droplets by interfacial tension (Fig. 1i–p). Explanations are partly based on the theory of viscoelastic phase separation (Tanaka, 1994
Tanaka, H. (1994) Critical dynamics and phase-separation kinetics in dynamically asymmetric binary fluids: New dynamic universality class for polymer mixtures or dynamic crossover? The Journal of Chemical Physics 100, 5323.
).